Q22 impulse at pt. P
Q23 impulse in string
Yesterday Philip was frustrated at the lack of gud problems...I am posting some for everyones practice..........2009 aspirants should solve them and put up solutions here for 2010 students to learn.............
Some might be easy.......but all are conceptual[1][1].....
Q1 A uniform rod of length 2a and mass m is rotating in horz plane about a smooth fixed pivot through the centre of rod with a constant angular velocity w rad/s.A particle of same mass moving with speed aw/4 opposite t orod strikes one end of the rod perpendicular to it.If e=1/2,calculate angular velocity of rod immediately after collision.
If anyone has problem with my initiative he/she is free to express his/her views...........i wont feel bad at all and i will stop this work at that moment only..[1][1][1][1]
-
UP 0 DOWN 0 11 144

144 Answers
Q23 .. v of m when it has fallen a height 8r = √16rg = 4√rg
let T be inpulse force acting for time dt
So -∫Tdt = mv - m4√rg
and r∫Tdt = 2m*r2/2*v/r
==> ∫Tdt = mv
So, 2mv = m4√rg
==> v = 2√rg
==> ω=2√g/r
and impulse of tension = ∫Tdt = mv = 2m√rg
8mgr = 1/2mv^2
m√16gr = P
mv - p = -J
Jr = mr^2 W ....
J = mWr ..
J = mv ...........
2mv = p
v = 2√gr
J = 2m√2gr???????????????[tukka\]
sorry last step mai galti ho gayi .............2m√gr ......root ke andar 2 nahin hai .............pata nahin kyun kiya ase..[3]
yep ashish is rite ..............
let it provide an impulse J ........
mv0R = JR ..
J= mv0 ....
simple .. more simple than urs.. iit:
as the state of motion of the disc is same before and after collision so the impulse provided by the pivot = mv0 and as there is no other force at P therefore impulse at P = mv0
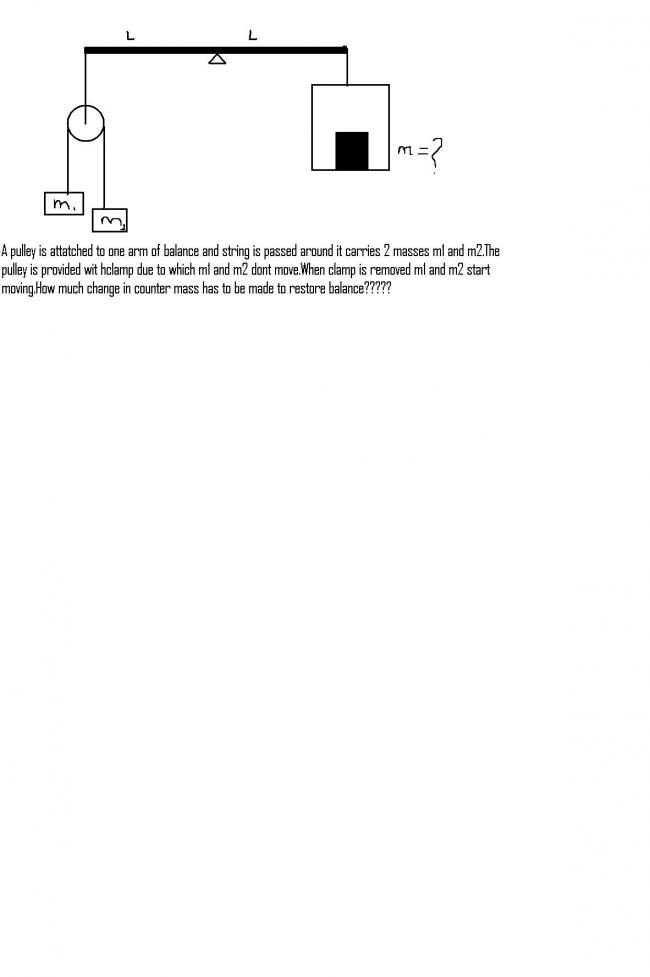
(m1-m2)g = (m1+m2)a
a= (m1-m2)g/(m1+m2) ...........
m1g - T = m1a
m1g - m1a = T
m1g(m2+m1 - m1 + m2 /m1+m2) = T
2m1m2g/(m1+m2) = T ........
2T = mg
4m1m2g/(m1+m2) = mg
4m1m2/(m1+m2) = m//
Thread restarted..Hoping to get same response as last time
Q25 ABC is a triangular framework of 3 uniform rods of mass m and length 2l.It is free to rotate in its own plane about smooth horz. axis through A which is perpendicular to ABC.If it is released from rest when AB is horizontal and C is above AB.Find max velocity of C in the subsequent motion
i think Q4 reqires a little more disscussion.....what i found that there will be a frictional torque when the ball strikes the edge of the ruler.....so angular momentum is only conserved at that point!!!!
Sorry for such a late reply..[2]
ur soln is rite philip
@grandmaster....we can discuss Q4..but tell ur dbt first
Here is new ques::
Q26 A disc of radius R and mass m is mounted on vertical axis through its centre and perpendicular to its plane.A rat of mass m runs around the rim of the disc with speed v anticlockwise relative to the stationary observer.The disc rotates clockwise with respect to stationary observer.The disc rotates clockwise with angular speed ω .The rat finds a piece of bread on rim ans stops.Find angular velocity of disc after rat stops
conserving the total angular momentum about the axis since friction b/w the rat and disc will be an internal force
mvR-\frac{mR^2}{2}w = \frac{3}{2}mR^2w_f \\\Rightarrow \frac{2v}{3R}-\frac{w}{3}=w_f
in the anticlockwise sense.
Q27 A small body A is fixed to inner side of thin rigid hoop of radius R and mass equal to half of body A.The hoop rolls without slipping over horz plane.At the moment when body A gets into lower position,the centre of hoop moves with velocity v.At what value of v,will the hoop moves without bouncing
Q27.
1.Energy conservation.
mv2/2+mv2R2/2R2=mv12/2+mv12R2/2R2 +mgR +m(2v1)2/4
(where v1 is the vel. at the topmost point.)
v2=gR+2v12
2.If N is the normal reaction exerted by the grnd on the hoop,N1 exerted by the hoop on the body,
N+N1=mg/2
In the balancing condition,N=0.
N1=mg/2.....
mg/2+N1=mv12/2R
v1=√2gr
thus,V=√5gr
I1*W1+I2*W2=I1W+I2W 3WE HAVE TO FIND TWO EQUATION
M*(2A)*(2A)/12+M*AW/4*A=M*(2A)*(2A)/12*W+MAV1
V2-V1=-1/2(WA-AW/4)SOLVE TWO FIND ANSWER
Sorry to all..I have bee na bit unfair to this thread....from now on i iwll update regularly....
@rahul,ans is √8gR