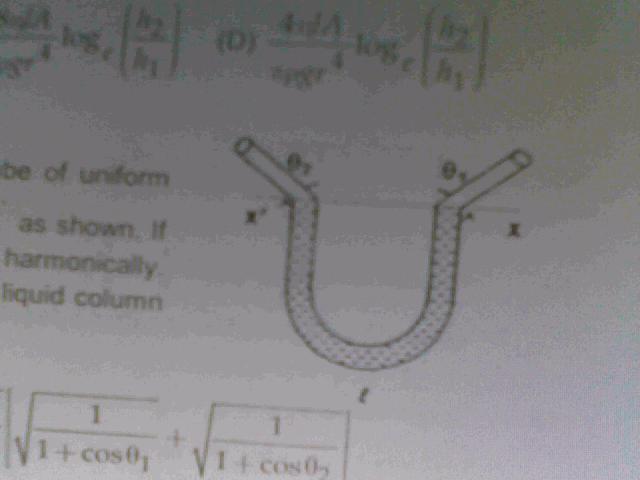
Here the SHM takes place with one frequency when the liquid rises through the right limb and with another frequency when it rises through the left limb.
Let's find the time taken for the liquid to move from the mean position, rise through the right limb and back to mean position (i.e. half the time period for the oscillation)
Now, since both open surfaces of the liquid is at atmospheric pressure, we can omit the effect of pressure in our analysis.
Let the liquid get displaced by x. Then the potential energy of the system is
\underbrace{\frac{\rho A(l-x)^2g}{2}}_{\text{for the liquid in the left limb}} + \underbrace{\frac{\rho A l^2g}{2}}_{\text{right limb vertical portion}} + \underbrace{\frac{\rho Ax(l+\frac{x \cos \theta_1}{2})g}{2}}_{\text{right limb angled portion}}
And the kinetic energy is \frac{1}{2} \times (2 \rho lAv^2)
So the total mechanical energy is:
E=\frac{\rho A(l-x)^2g}{2} + \frac{\rho A l^2g}{2} + \frac{\rho Ax(l+\frac{x \cos \theta_1}{2})g}{2} + \frac{1}{2} \times (2 \rho lAv^2)
Since it is a constant we have
\frac{dE}{dt}=0
which yields
a =- \frac{g(1+\cos \theta_1)x}{l}
Now, its easy to see that the time period of motion is
\pi \sqrt{\frac{l}{g}} \left[\frac{1}{\sqrt{1+\cos \theta_1}} + \frac{1}{\sqrt {1+\cos\theta_2}} \right]