General Theory of Relativity

Equivalence Priciple

The newly discovered relativity of space and time was very important in Einstein's desire to understand gravity. Einstein found Newton's description of gravity disappointing, because it assumes that space and time are absolute. However, gravity causes a falling object to accelerate and, according to the special theory of relativity, the resulting motion also affects rulers and clocks that are moving with the moving object. Thus, Einstein argued that gravity must affect the shape of space and the flow of time.

To explain how gravity can behave in this way, Einstein began by demonstrating that it is not necessary to think of gravity as a force. According to Newton's theory, an apple falls to the floor because of the force of gravity pulls the apple down. Einstein pointed out that the apple would appear to behave in exactly the same way in space, far from the Earth's gravity, if the floor were to accelerate upwards. In other words, the floor comes up to meet the apple.

In the figure(shown on the right), the famous two gentlemen are watching an apple fall towards the floor of their compartments. They have no way of telling who is at rest on the Earth and who is in the hypothetical elevator moving upward at a constantly increasing speed. This is an example of Einstein's principle of equivalence, which states that in a small volume of space, the downward pull of gravity can be accurately and completely duplicated by an upward acceleration of the observer. The principal of equivalence allowed Einstein to focus entirely on motion, rather than force, in discussing gravity. Far from a source of gravity, a falling body has only a small acceleration, so the effect on clocks and rulers moving with the body is small. Nearer a source of gravity, the falling body has a larger acceleration, so the distortion of clocks and rulers is larger as well. In this way, Einstein "generalized" his special theory to arrive at his general theory of relativity, published in 1915.

Curvature of Space

A hallmark of gravity is that is causes the same acceleration no matter what the mass of the object. For example, a baseball and a cannon ball have very different masses, but if you drop them side-by-side, they accelerate downward at exactly the same rate. To explain this, Einstein envisioned gravity as being caused by a curvature of space. In fact, his general theory of relativity describes gravity entirely in terms of the geometry of both space and time. Far from a source of gravity, like a planet or a star, space is "flat" and clocks tick at their normal rate. Closer to a source of gravity, however, clocks slow down and space is curved. A useful analogy is to imagine that the space near a massive object such as the Sun becomes curved like a surface in figure.

Imagine a ball rolling along this surface. Far from the "well" that represents the Sun, the ball would move in straight line since the surface is fairly flat. If it passes the well, however, it would curve in toward the well. If it is moving at an appropriate speed with respect to the well, it might move in an orbit aroundthe sides of the well.In this analogy,it is the curvatureof the surface that makes the ball follow a curved path near the well. The curvature has the same effect on a ball of any size, which explains why gravity produces the same acceleration on objects of different mass.

Orbit Precession

One of the first things Einstein did with his new theory was to calculate the orbits of the planets. Einstein realized that if his theory was correct, it should be able to predict accurately the well-known motions of the planets about the Sun. According to general relativity, space far from the Sun is almost flat and objects thus travel along nearly straight-line paths. Near the Sun, planets and comets travel along curved paths because space itself is curved. Einstein found that where gravity is weak, the general theory of relativity gives exactly the same results as the classical theory of Newton. But in stronger gravity, such as is found very near the Sun's surface, the general theory of relativity predicts that there will be noticeable effects upon space and time, and the Newtonian theory of gravity is no longer accurate.

Even before Einstein, scientists knew that the motions of the planets closest to the Sun do not agree with Newtonian mechanics. During the mid-1800s, French astronomer Urbain Le Verrier (famous for his prediction of a planet beyond Uranus) pointed out that Mercury was not following its predicted orbit. As the planet moves along its elliptical orbit, the orbit itself rotates or processes.

As shown in Figure, the long (major) axis of Mercury's orbit slowly changes orientation. (Somewhat confusing, the term precession is also used to describe how the axis of rotation of a top or a planet changes direction. Most of the Mercury's precession is caused by the gravitational pull of the other planets, as explained by Newtonian mechanics. But once the effects of all the other planets had been accounted for, there remained an unexplained excess rotation of Mercury's major axis of 43 arcsec per century. Although this discrepancy may seem small, it frustrated astronomers for half a century. Some astronomers even searched for a missing planet even closer to the Sun that might be tugging on Mercury; none has ever been found. But Einstein showed that his theory could account for the excess precession of Mercury's orbit. It was a spectacular confirmation of his general theory of relativity.

Deflection of light

To help validate his theory, Einstein made other predictions that could be tested. With his calculations he showed that light rays passing near the surface of the Sun should appear to be deflected from their straight-line paths because the space through which they are moving is curved. In other words, gravity would bend light rays, an effect not predicted by Newtonian mechanics because light has no mass.

Figure above shows a beam of light from a star passing by the Sun and continuing on to the Earth. Because the light ray is bent, the star appears to be shifted from its actual location. The largest deflection (a mere 1.75 arcsec) occurs for light rays grazing the Sun's surface.
This prediction was first tested in 1919 during a total solar eclipse. During the precious moments of totality, when the Moon blocked out the blinding solar disk, astronomers succeeded in photographing the stars around the Sun. Careful measurements afterward revealed that the stars were shifted from their usual positions by an amount consistent with Einstein's theory. General relativity had passed another important test.

Redshift

Einstein also made a third prediction. Stated that because gravity causes time to slow down, clocks on the ground floor of a building would tick more slowly than clocks on the top floor, which are farther from the Earth (Figure a). A light wave can be thought of as a clock; just as a clock makes a steady number of ticks per minute, an observer sees a steady number of complete cycles of light wave passing by each second. Hence, if a light beam is aimed from the ground floor to the top floor of a building, an observer at the top floor will measure the light to have a lower frequency, and thus a longer wavelength, than an observer on the ground floor (Figure b). Because an increase in wavelength is a red shift, this effect is called the gravitational red shift.

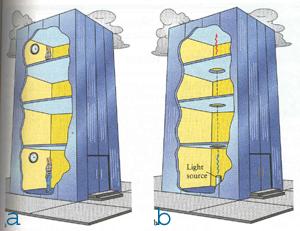
The American physicists Robert Pound and Glen Rebka first measured this effect in 1960 using gamma rays fired between the top and bottom of a shaft 20 meters tall. Because the Earth's gravity is relatively weak, they measured only a very small red shift (INSERT FORMULA), but this value was in complete agreement with Einstein's prediction Much larger gravitational red shifts are seen in the spectra of white dwarfs, whose spectral lines are red shifted as light climbs out of the white dwarf's intense surface gravity. As an example, the gravitational red shift of the spectral lines of the white dwarf Sirius B is (INSERT FORMULA), which again agrees with the general theory of relativity.
Be careful not to confuse the gravitational red shift with a Doppler shift. In the Doppler effect, red shifts are caused by a light source moving away from an observer. Gravitational red shifts, by contrast, are caused by time flowing at different rates at different locations. No motion is involved