9
5 Answers
1yup me too was getting the same pattern but it doesnt prove it thats the problem ..
1well i really didnt try it thats the problem .. once tried got a solution ..
by induction :)
we have found out that the first tern has to be 1 .. other wise the same will repeat so let it be 1
our conjecture = an=n
so let upto k terms we have ak=k
so we get
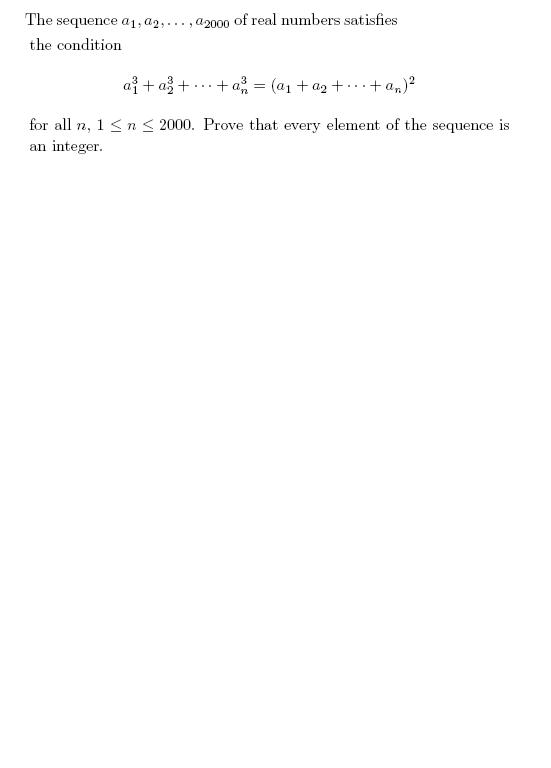
a13+a23+....ak3=(a1 +a2+...ak)2
a13+a23+....a(k+1)3=(a1+a2+...ak+1)2
subtract these ..
we get
a(k+1)=a(k+1)(2S+a(k+1)) S= sum of first k terms
ak+1=0 is one solution but the sequence then shall start all over again .. so we neglect it
another two we get from the quadratic as
ak+1 = (1±√(8S+1))/2
we know S=1+2+...k=k(k+1)/2
sop we get 8S+1 as (2k+1)2
so we get ak+1 as -k or k+1
if ak+1=-k then
the ak and ak+1 cancel out and we start again from ak-1 .. thus we take ak+1=k+1 and
thus get the solution
9but that gives the proof rohan
ie all nos are integers
possible patterns acc to post 2 are
123456789......
0000000000......
or a mixture of the above two patterns(preceded by nos of form k,-k) as in
123-3(34567 ..... )
123-3(0000003456...)
ans is quite trivial by observation
