30This is the question after which Solid State becomes a topic that you love!
We know an unit cell is something that has all the characters of the entire solid.. or in other words, it is the prototype for the entire solid..
We also know that in CCP we observe ABC-ABC layering..
Can you show ABC-ABC layering in an unit cell of CCP?
-
UP 0 DOWN 0 0 16
16 Answers
3030atleast I have.. You can't imagine how!
Using paachak lol. [3]
49:P
I did using paint! :P
Which brand of pachak by the way? [6]
30I don't know.. It was homemade I guess. Perfectly spherical ones. I tell you what an experience it was.
A tougher question is to find the voids in an HCP unit cell!
1i knw the 2nd qstn as well....i did the 1st qstn wid the help of ice blocks n i`m nt joking
rajiv sir explained this to us as well
49maybe describing the layers??
I will try to re-create the picture in paint! :P
30I just tried doing it. Its better (for the sake of others) to get a standard image.
As for the explanation part, the thing is to look down the diagonal.
We can see a traingle form if we join the face diagonal of any face, the adjacent face centres and the bottom - most sphere common to the adjacent faces. This forms layer A
Now, exactly opposite layer can be formed if other three faces are considered, and the triangle is considered upside down. Forms layer B with spheres in the tetrahedral voids of A.
Now, only two vertex spheres are left. These can be considered as the C layer atoms!
Did that make sense?
30This should be it :
Subho da don't kill me for this! I couldn't control the urge! :D :P
49I finally managed to get a understandable picture for your explanation using paint:
omg what a mind-boggling diagram was that Ashish! :P
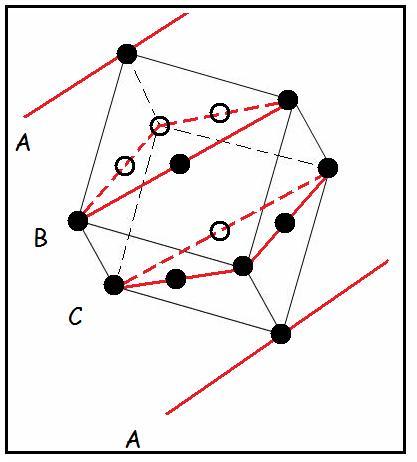
makes sense??
maybe from a different angle??
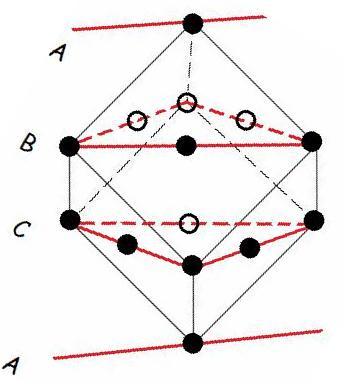
clear? [4]
30Unbelievable! [240]
I never thought this could come out of paint!
WOW! :D
30By the way , I hope the second question is still on! not many seem to be interested in this :(
"Find the voids in an HCP unit cell."
1http://upload.wikimedia.org/wikipedia/commons/8/86/FCC_primative-cubic_cells.svg