24Anyways answer remians same..becoz answer didnt depend on that term (L→∞)[3]
Suppose that a bubble has the shape of a long cylinder, rather than that of a sphere. Determine an expression for the difference between the inside and outside pressures; express your answer in terms of the surface tension γ and the radius R of the cylinder
PS: Solving this question explains a lot of very basic things about surface tension :) .. Do not ignore ;)
Source: Internet...
-
UP 0 DOWN 0 1 18
18 Answers
3@eure that i know ......ur looking only at the force coused by T in that interface .... that 2r is part of the actual surface of bubble .. there is no proj here .....
24ohh...ya i took only one side of cylinder..
so I just need to multiply my result with 2 naa ??
62The answer will be like this..
You have all made some slight mistakes..
Eureka has taken only one film...
WHile IITimcomin has made a mistake of taking force due to pressure as P(2piRL) The logic of which is nto clear to me..
According to me the method should be
Pi-Po(2RL) = T(2L+2L+4r+4R)
So Pi-Po = T (2L+4R) / LR = 2T/R + 4T/L
since L is very large, it is same as 2T/R
(Here I have assumed pure cyllindrical shape which may not be ture.. but it wont matter because the lenght is very large in comparison to the radius...)
24
1@eureka surface tenion is like kind of internal force in between the two halves.......so it go to act over the perimeter of the rectangle ......:)
3xyz he said ur ans is rong cuz u didnt take L>>R... else it shud be right!!!!
24@xyz
Tension force wont act on projection.....it will act on pure bubble surface
so pure bubble surface will have perimeter...Ï€R+Ï€R+2L
62are you sure karna... (It is given that L is very large... )
So the answer will be independent of L
Try again.. (not that this needs to be necessariliy true)
1@eureka how are u taking the perimeter to be 2pir+2l.........
the cross section will be a rectangle of dimensions 2r and l
corect me if i am wrong
24@ iitimcomin
Tension force wont act on projection.....it will act on pure bubble surface
@xyz
ur terms are diff
1even i have done the same thing in first post...but nishant sir has told that its wrong
3eure u made a mistake...
it should be
T(2(2R+L))..........its the perimeter of the recangle u get as crossection.... think abt it.....
so taking R<<L u get T/R..........[FOR LIQUID]........
so for soaP... u get 2T/R!!!
#6 is another way f doin it u get same ans...[1]
24Cutting the cylinder through middle
Pout(2RL)+T(Ï€R+Ï€R+2L)=P(2RL)
=>(P-Pout)=T(2Ï€R+2L)2RL
=>(P-Pout)=T(Ï€R+L)RL
=>(P-Pout)=T[Ï€L+1R]
L→∞
=>(P-Pout)=TR
Correct soln
Pout(2RL)+T(2R+2R+2L)=P(2RL)
=>(P-Pout)=T(4R+2L)2RL
=>(P-Pout)=T(2R+L)RL
=>(P-Pout)=T[2L+1R]
L→∞
=>(P-Pout)=TR
also edit :
Put γ in palce of T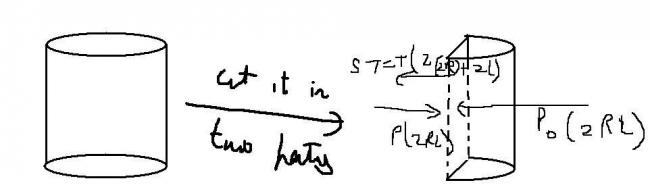
3me getting Pex=2Y/R
Since the L>>>R
we have Y.(πLΔr+πΔLR)=(ΔP).(2ΠLrΔr+πΔLR)
which yields us ΔP=2Y/r
3let there be excess pressure .P ... inside .....
therefore .. we have ..
P(2piRL)dr = 2T(2piLdr + 2piRdr)
P = 2T(L+R)/(RL)
if L>>R
we have P = 2T/R