WOW!
kaymant sir,
Are these types of assumptions/transformations available in some book?
Solve this integral
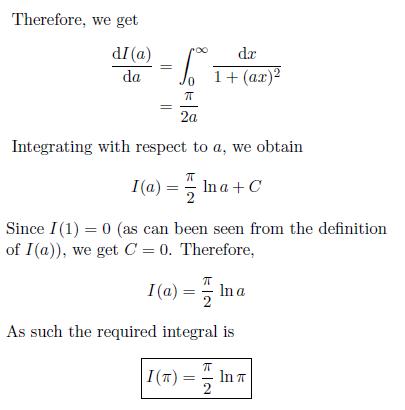
\int_{0}^{infinity}{\frac{tan^{-1}(\pi x)-tan^{-1}x}{x}}dx
I don't know why but my latex tool is not working (its not able to copy the things into the editor), so I typed the solution on my system and am uploading as image).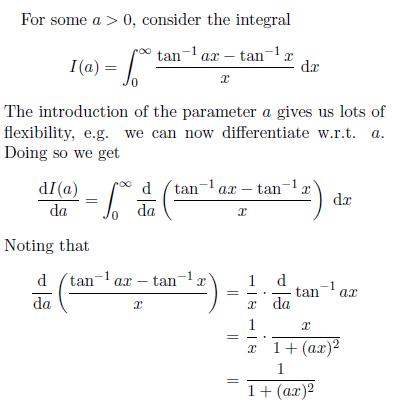
WOW!
kaymant sir,
Are these types of assumptions/transformations available in some book?
Well these tricks are (sadly) not available in any introductory text to integral calculus.. however you may always introduce a parameter and then the integral will open a wide range of interesting subresults obtained by many transformations. Differentiation is one of those tricks but that's not the only one. I am afraid, any reference that I'll suggest will be beyond your reach presently.
Having said that, if you like you can look for a book called "Irresistible Integrals" by George Boros and Victor H. Moll. But mind you, the book is quite advanced.
Ok[1]
But can you tell us some of those tricks other than differentiation which are useful for JEE ?
Kaymant Sir i have a doubt sir wen u differentiated I(a) u only differentiated the part inside the integral usual then we defferentiate a integral we open it like F(x) = b(x)∫a(x)g(x)dx
dF(x)/dx = g(a) a`(x) - g(b)b`(x) i am rite sir?
Then here why did u only differentiate the inside part
The differentiation you are talking about is carried when the limits are variable. In this case I am not doing so. Rather, the right hand side will have a value which will depend on the parameter a. So I can take it as s function of a. Further, while differentiating w.r.t. a, I have interchanged the order of differentiation and integration. This is entirely justified.