1is it usin vectors?ie cross pdt?if itz so then i can prove
9 Answers
1if u r familiar with the proof of cos(a-b) then u can use this....
sin(a+b)=cos(Î /2-(a+b))
=cos((Î /2-a)-b)
=coa(Î /2-a)cosb+sin(Î /2-a)sinb
=sin a cos b+sin b cos a
62no dear .. i am looking for a fundamental proof.. not this one..
i mean if u are using this result of cos (a-b) prove that!
I hope u understand what i am saying :)
1I can prove that in two diff ways .........
is it fine , if I put it 2moro.
I got ur point..........
33
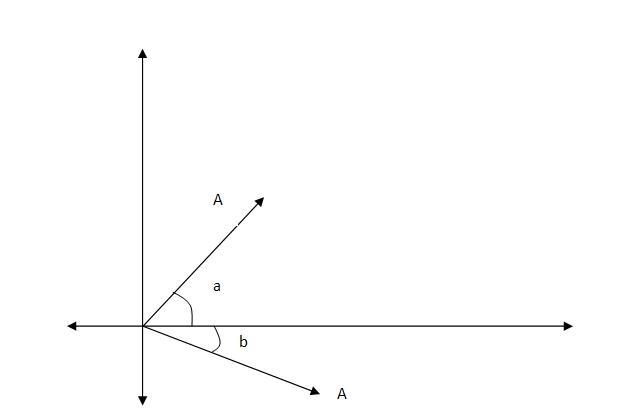
Both vectors have equal magnitude A
|A1XA2| by components.....
gives...
A2(cosa.sinb+sina.cosb)..........................(i)
now cross product by A2sin(a+b) ...............(AB sinθ)...........(ii)
from (i) and (ii) sin(a+b)=(cosa.sinb+sina.cosb).
..................................................................................................