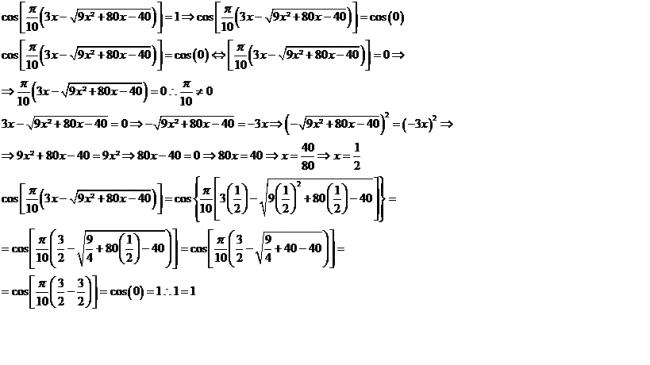71Writing right side as cos 2nπ n ε I , then Solving,
I'm getting x = 2 n + 15 n
So for any Integer n, How is there any Integral Solution to above?
Sorry If I', wrong!
\hspace{-16}$Find all Integer Roots of the equation\\\\ $\mathbf{\cos\left(\frac{\pi}{10}\left(3x-\sqrt{9x^2+80x-40}\right)\right)=1}$
-
UP 0 DOWN 0 0 3
3 Answers
71
71harold. It is not correct I think.
The more general solution from Wolfram.
The method is still the same as I said earlier My workings might have been wrong. Because only that would infuse ' n ' into equation.
